The papers below all precede the finding that tauquernions map to SO(4) and tauquinions to SU(3), whence it is assured that the model being presented here has the overall structure U(1) x SU(2) x SU(3) x SO(4), ie. the Standard Model of quantum mechanics augmented with relativistic 3+1d spacetime.
The term “tauquernion” derives from the mathematical term “quaternion”, in part to retain the mnemonic ring of “quaternion”, and as well because “tau” itself often designates a time variable. Both apply.
Do you know what “quaternions” are? [Full explanations are in the papers!]
They are a different phase-based way to describe the axes of 3-space, using outer-product vector multiplication
. The unit quaternions are the three mutually perpendicular planes
. MORE: Each of these squares mathematically to -1 : taking
, so each is a representation of
.
‘s powers cycle through the four quadrants,
, exhibiting the sequence of phases that determine an entity’s coordinates. In the end, an
coordinate is turned into
, where
are the powers of
that determine which quadrant (4 in the plane, 8 in 3-space).
It follows that motions in the quaternion coordinate system are reversible, since each quaternion is a root of unity.
They are called quaternions, with the connotation of 4-ness, because their discoverer, Hamilton, saw that when combined with a (fourth, scalar) time coordinate, he had discovered a brand new way to look at 3d space and its relationship to time, namely that the two domains are perpendicular to each other, via
: take space as “imaginary” and time as “real”. In this 4-d sense, quaternions are widely used in the theory of special relativity. In quantum mechanics, as here, the phase/wave aspect dominates and time t is confined to irreversible multiplications (= energy use and entropy creation).
So back to tauquernions. They are exactly like quaternions except that they are not reversible. That is, particulate motion in tauquernion space always costs energy. And basically, energy use = time’s tick. This is another way to describe the Higgs field – the creator of gravity, mass, space, time, and entropy – and that is what the following paper does:
TauQuernions 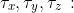
3+1 Dissipative Space out of Quantum Mechanics
The tauquernions are inhabitants of the geometric (Clifford) algebra Noting that
is isomorphic to the Pauli algebra, tauquernions therefore fall outside of the Standard Model of quantum mechanics (a good thing here).
It turns out that the sum has exactly the properties of the Higgs boson. Simultaneously,
are themselves ebits, ie. entangled EPR information, and so the mechanism underlying space, mass, gravity and entropy turns out to be entanglement! All this and more is described in the above paper. BTW, one referee report described the mathematics as “sound” and “of interest in its own right”, so just relax and enjoy!
The algebraic theory of computational processes applied in the preceding paper is described in:
Synchronization: The Font of Physical Structure
This paper, aka. SynchFont, analyzes the computational concept of inter-process synchronization in the form of the two primitive operations wait(event) and signal(event). It turns out that waits correspond to bosons and signals to fermions. All very clean and completely in accord with quantum measurement theory. So this establishes a solid, and novel, bridge between computation and quantum physics.
The following is a lighter piece, but in preceding the TauQuernion paper by several years, the result that comes out of plugging our geometric algebra into the Riemann Hypothesis is clearly prescient in its characterization of distributed systems. The process-representation of SynchFont shows up in the context of wave-particle duality, among a number of other observations of interest to those interested in process-based (Heracletian) systems.
Leave a Reply